See how the equation form of Ohm's law relates to a simple circuit. Adjust the voltage and resistance, and see the current change according to Ohm's law. The sizes of the symbols in the equation change to match the circuit diagram.

See how the equation form of Ohm's law relates to a simple circuit. Adjust the voltage and resistance, and see the current change according to Ohm's law. The sizes of the symbols in the equation change to match the circuit diagram.

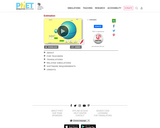
This interactive Flash animation allows students to explore size estimation in one, two and three dimensions. Multiple levels of difficulty allow for progressive skill improvement. In the simplest level, users estimate the number of small line segments that can fit into a larger line segment. Intermediate and advanced levels offer feature games that explore area of rectangles and circles, and volume of spheres and cubes. Related lesson plans and student guides are available for middle school and high school classroom instruction. Editor's Note: When the linear dimensions of an object change by some factor, its area and volume change disproportionately: area in proportion to the square of the factor and volume in proportion to its cube. This concept is the subject of entrenched misconception among many adults. This game-like simulation allows kids to use spatial reasoning, rather than formulas, to construct geometric sense of area and volume. This is part of a larger collection developed by the Physics Education Technology project (PhET).

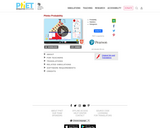
The students will play a classic game from a popular show. Through this they will see the probabilty that the ball will land each of the numbers with more accurate results coming from repeated testing.

Learn about the physics of resistance in a wire. Change its resistivity, length, and area to see how they affect the wire's resistance. The sizes of the symbols in the equation change along with the diagram of a wire.

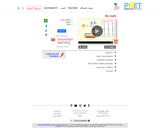
Learn about the physics of resistance in a wire. Change its resistivity, length, and area to see how they affect the wire's resistance. The sizes of the symbols in the equation change along with the diagram of a wire.

Students learn how different characteristics of shapes—side lengths, perimeter and area—change when the shapes are scaled, either enlarged or reduced. Student pairs conduct a “scaling investigation” to measure and calculate shape dimensions (rectangle, quarter circle, triangle; lengths, perimeters, areas) from a bedroom floorplan provided at three scales. They analyze their data to notice the mathematical relationships that hold true during the scaling process. They see how this can be useful in real-world situations like when engineers design wearable or implantable biosensors. This prepares students for the associated activity in which they use this knowledge to help them reduce or enlarge their drawings as part of the process of designing their own wearables products. Pre/post-activity quizzes, a worksheet and wrap-up concepts handout are provided.

Build coin expressions, then exchange them for variable expressions. Simplify and evaluate expressions until you are ready to test your understanding of equivalent expressions in the game!

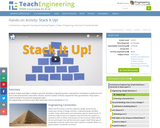
Students analyze and begin to design a pyramid. Working in engineering teams, they perform calculations to determine the area of the pyramid base, stone block volumes, and the number of blocks required for their pyramid base. They make a scaled drawing of the pyramid using graph paper.

This learning video presents an introduction to graph theory through two fun, puzzle-like problems: ''The Seven Bridges of Konigsberg'' and ''The Chinese Postman Problem''. Any high school student in a college-preparatory math class should be able to participate in this lesson. Materials needed include: pen and paper for the students; if possible, printed-out copies of the graphs and image that are used in the module; and a blackboard or equivalent. During this video lesson, students will learn graph theory by finding a route through a city/town/village without crossing the same path twice. They will also learn to determine the length of the shortest route that covers all the roads in a city/town/village. To achieve these two learning objectives, they will use nodes and arcs to create a graph and represent a real problem.

Learn how to add vectors. Drag vectors onto a graph, change their length and angle, and sum them together. The magnitude, angle, and components of each vector can be displayed in several formats.

Students apply their knowledge of scale and geometry to design wearables that would help people in their daily lives, perhaps for medical reasons or convenience. Like engineers, student teams follow the steps of the design process, to research the wearable technology field (watching online videos and conducting online research), brainstorm a need that supports some aspect of human life, imagine their own unique designs, and then sketch prototypes (using Paint®). They compare the drawn prototype size to its intended real-life, manufactured size, determining estimated length and width dimensions, determining the scale factor, and the resulting difference in areas. After considering real-world safety concerns relevant to wearables (news article) and getting preliminary user feedback (peer critique), they adjust their drawn designs for improvement. To conclude, they recap their work in short class presentations.